Báo cáo khoa học: "Branchiness of Norway spruce in north-eastern France: modelling vertical trends in maximum nodal branch size"

Chia sẻ: Nguyễn Minh Thắng | Ngày: | Loại File: PDF | Số trang:15

Thêm vào BST

Báo xấu

32
lượt xem 3
download

Download Vui lòng tải xuống để xem tài liệu đầy đủ

Tuyển tập các báo cáo nghiên cứu về lâm nghiệp được đăng trên tạp chí lâm nghiệp quốc tế đề tài: "Branchiness of Norway spruce in north-eastern France: modelling vertical trends in maximum nodal branch size...

Chủ đề:

Bình luận(0) Đăng nhập để gửi bình luận!

Lưu

Nội dung Text: Báo cáo khoa học: "Branchiness of Norway spruce in north-eastern France: modelling vertical trends in maximum nodal branch size"

Original article Branchiness of Norway spruce in north-eastern France: modelling vertical trends in maximum nodal branch size F Colin F Houllier 1 INRA, Centre de Recherches Forestières de Nancy, Station de Recherches sur la Qualité des Bois, 54280 Champenoux; 2 ENGREF, Laboratoire ENGREF/INRA de Recherches en Sciences Forestières, Unité Dynamique des Systèmes Forestiers, 14, rue Girardet, 54042 Nancy Cedex, France (Received 13 March 1991; accepted 12 September 1991) Summary — This paper is part of a study which aims at proposing a new method for assessing the wood quality of Norway spruce from northeastern France. One component of this method is a wood quality simulation software that requires detailed inputs describing tree branchiness and morpholo- gy. The specific purpose of this paper is to present a model that predicts maximum limbsize at vari- ous points along the stem. The dependent variable of the model is the maximum diameter per annu- al growth unit. The independent variables are the relative distance from the growth unit to the top of the stem and some combinations of standard whole-tree measurements and general crown descrip- tors. The equation is a segmented polynomial with a join point at the height of the largest branch di- ameter for each tree. First, individual models are fitted to each sample tree. Then a general equation is derived by exploring the behaviour of the individual tree parameters of the polynomial model as functions of other individual tree attributes. Finally the model is validated on an independent data set and is discussed with respect to biological and methodological aspects and to possible applications. branchiness / crown ratio / modelling / wood resource / wood quality / Picea abies Résumé — Branchaison de l’épicéa commun dans le Nord-Est de la France : modélisation du diamètre maximal des branches verticillaires le long de la tige. Cet article s’insère dans un pro- jet qui vise à proposer une méthode d’évaluation de la qualité de la ressource en épicéa commun du Nord-Est de la France. Ce projet s’appuie notamment sur un logiciel de simulation de la qualité des sciages (Leban et Duchanois, 1990) qui nécessite une description détaillée de la morphologie et de la branchaison de chaque arbre. Cet article a pour but de proposer un modèle de prédiction de la distribution du diamètre des branches le long de la tige. La variable prédite est le diamètre maximal de branche par unité annuelle de croissance. Les variables indépendantes du modèle sont la dis- tance de l’unité de croissance à l’apex ainsi que des combinaisons des variables dendrométriques usuelles et des descripteurs globaux du houppier. L’équation est non linéaire et segmentée autour d’une valeur critique qui correspond à la position de la plus grosse branche de l’arbre. On ajuste d’abord un modèle individuel pour chaque arbre échantillonné. Puis on construit un modèle global à partir d’une analyse du comportement des paramètres du modèle individuel en fonction d’autres ca- ractéristiques dendrométriques. Ce modèle est ensuite validé sur un jeu de données indépendantes. On discute finalement des propriétés de ce modèle tant au plan méthodologique et biologique qu’au plan de ses possibilités d’utilisation. branchaison / houppier / modélisation / ressource en bols / qualité du bols / Picea abies
INTRODUCTION This sofware and the results of the present study will be integrated into a sys- tem for predicting the quality of the conifer- Description and modelling of tree branchi- ous wood resources from the data record- may be carried out in various con- ness ed by regional or national forest growth and yield investigations, silvi- texts: inventories. This project deals specifically cultural and genetic experiments, logging with Norway spruce in northeastern France and wood quality studies. The analysis (ENGREF, INRA, UCBL, 1990). and the prediction of branch size (ie Until now the project has focused on branch diameter) is obviously one of the mid-size with a diameter at breast height most important features of branchiness (DBH) ranging between 15 and 35 cm. studies. Several authors have already con- There are 2 reasons for this choice: 1), this sidered the limbsize at various heights: size range will provide most of the stems Madsen et al (1978), at 2.5, 5 and 7.5 m that will be harvested in the coming dec- from ground level; Hakkila et al (1972), at ades; 2), the prediction of the quality of 70% of the total height, De Champs these logs is important because they may (1989), at the fourth and eighth whorl either be sawn or utilized as pulpwood. counted from tree base; Maguire and Applications of this study are not limited Hann (1987), at the point where the radial to this particular project, since branching extension of the crown is at its maximum. structure can also be related to growth Other authors (Ager et al (1964) and modelling. Indeed, crown development and Western (1971) in Kärkkaïnen (1972) op recession are intimately linked to wood cit; Kärkkäinen (1972), Uusvaara (1985)) yield through the interactions between observed the relationship between limb branch size, leaf area and carbon assimila- size and the distance from the top of the tion rate. Therefore, information on branch stem. However, few studies have tried to size at various stages of stand develop- model this vertical trend and predict the ment provide an insight into the dynamic maximum limbsize anywhere along the interactions between stem and crown. (Maguire et al, 1990, on Douglas fir). stem This study aims to develop a limbsize MATERIAL AND METHODS links standard whole-tree that model measurements (age, total height, diameter at breast height) to the required inputs of a Study area wood quality simulation software (Simqua; Leban and Duchanois, 1990). This soft- All the trees were sampled in the Vosges depart- ware requires information on stem taper, ment, in the northeastern part of France where ring width patterns and branching structure Norway spruce stands are mostly located in the (insertion angle, diameter, number of no- Vosges mountains, at elevations ranging from dal and internodal branches). It can then 400 to 1 100 m. The approximate annual precipi- tation is between 800 and 2 200 mm while mean simulate the sawing process for any board temperature ranges from 8 to 5 °C. Snow is sawn from any stem for which this detailed abundant above 800-900 m. information is available. It can further sim- In the pre-Vosgian hills, sandstone with volt- ulate lumber grading by examination of the zite prevails on the western side, while much di- 4 faces of each board and application of versity appears (limestone, clay, sandstone) on grading rules (for instance, French grading the eastern side. The lower Vosges, between rules for softwood lumber). 350 and 900-1 000 m, are composed of triassic
the Statisti- definition of the latter parameter, limestones, which produce acid soils covered by see forests, and also permian limestones, which cal analysis section). yield richer soils that are seldom occupied by fo- high Vosges are composed of gran- rests. The ites of various kinds, producing primarily rich Data collection soils, although these soils can sometimes be poor to very poor (Jacamon, 1983). For the first 2 subsamples, the following vari- ables were measured: the length of each annual shoot and the dis- - Sampling tance from the top of the tree to the upper bud scale scars (measured to the nearest 2 cm); the diameter over bark for each whorl branch subsamples were collected, 2 for building Three - (ie having a diameter > 5 mm) with a digital cali- the model and the third one for its validation. per (to the nearest mm and at a distance from The trees of the 2 first subsamples were meas- the bole that was approximately equal to one ured after felling whereas the last subsample branch diameter); was obtained by climbing the trees. the "height to the live crown" which was de- - fined as the height from the base of the tree to Subsample 1 the first whorl including more than three- quarters of green branches (modified from Ma- sample trees (between 30 and 180 years of The guire and Hann, 1987, op cit); came from public forests managed by the age) the total height of the stem and the diameter - ONF (Office National des Forêts). In 1988, 10 at breast height; trees without severe damage from late frosts the age by counting the number of rings at the - and/or forest decline (in upper elevations) were stump after felling. sampled in 10 stands, for which the current den- For the third subsample, only the diameter of sity ranged between 500 and 1 500 stems per the thickest whorl branch, instead of the diame- ha. The past silviculture of these stands was un- ter of each whorl branch, was measured. known. Subsample 2 Statistical analysis In 1989, 16 trees were removed by thinning in a Two kinds of data were used: "the branch de- private experimental plantation, managed by AF- scriptors" and the "whole-tree descriptors". The OCEL (Association Forêt-Cellulose). This stand latter were the standard tree measurements and represents a fairly intensive silvicultural regime different crown heights and crown ratios: when compared with usual practices carried out AGE = total age of the tree (in years); in non experimental stands. The seedlings (6 DBH diameter (of the stem) at breast height = years in the nursery) were installed in 1961 and (in cm); then thinned in 1974, 1983 and 1989. H total height of the stem (in cm); = H/DBH = = ratio between H and DBH; Subsample 3 HFLB = height to the first live branch (in cm); HBLC = height to the base of the live crown as belonging to the first sub- For 9 of the 10 stands previously defined (in cm); sample, and for 7 trees in each of these stands, the diameter of the thickest whorl branch per an- HC average of the 2 previous heights, HFLB = nual shoot was collected up to the maximum and HBLC (in cm); height that it was possible to reach by climbing. 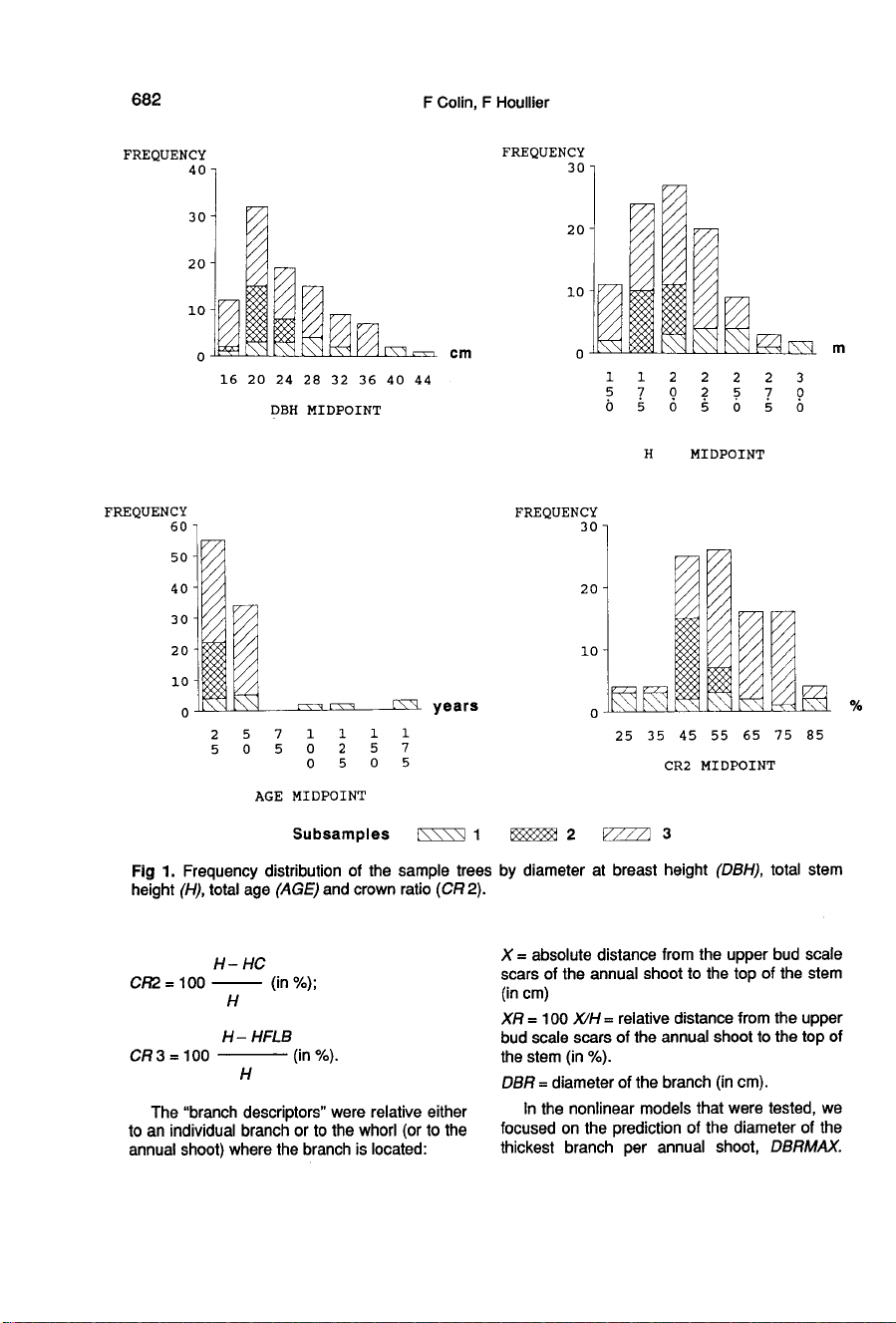Figure 1 shows the frequency of samples by diameter at breast height, total stem trees height, total age and crown ratio (for an exact
absolute distance from the upper bud scale X = of the annual shoot to the top of the stem scars (in cm) XR = 100 X/H = relative distance from the upper bud scale scars of the annual shoot to the top of the stem (in %). DBR = diameter of the branch (in cm). In the nonlinear models that were tested, we The "branch descriptors" were relative either focused on the prediction of the diameter of the to an individual branch or to the whorl (or to the thickest branch per annual shoot, DBRMAX. annual shoot) where the branch is located:
The variables (ie the predictors) independent RESULTS the whole-tree measurements as well as were the absolute and relative distances to the top 1 . The analysis was carried out in 4 steps: Individual models First step: We tried to model the variation of DBRMAX along each stem with individual equa- Several preliminary models were explored tions (one per tree) according to the relative dis- and tested. A modified Chapman-Richards tance to the top of the stem, XR : equation was one of the best: where i denotes the ith tree, jthe jth annual shoot,Θ the model parameters specific to the i (ie the differential form of the usual Chap- i th tree and &i,j random homoscedastic and non epsiv; man-Richards model with a, β and y be- autocorrelated variable. 0, β and &gam a; ≥ 1). ing parameters: a> Second step: We analyzed the variability of the parameters &i in relation to the whole tree de- Theta; However, it did adequately describe not scriptors and then tried to fit temporary equa- the peak of the experimental curve around tions of the following type: the thickest branches of the stem. Indeed, the prediction of the thickest branch of the g(DBH H AGE H CR 1CR 2 , , , /DBH iiii , ,, ii &iatehT ; = tree was not efficient, either for the location CR 3 (2) , , , ψ) i i i η HFLB HBLC HC +i , i of this branch along the stem or for its di- ameter. where ψ denotes the model parameters global to all trees and &i a random error. common eta; By observing the actual DBRMAX distri- Third step: We moved from the individual mod- bution along the stem, the idea was pro- els towards a global model by progressively re- posed to choose a segmented second or- placing the &i parameters in (1) by their predic- Theta; der polynomial model (Max and Burkhardt, tions (equation 2). We finally obtained models of 1975; Tomassone et al, 1983, p 119-122; the following form: with a join point value (ξ) which is the loca- i,j i,j i i i DBRMAX f(XR Θ(DBH H AGE ,,,, tion of the estimated thickest branch: = , /DBH ,...; i i psi;)) + ϵ H CR1 &i,j (3) These global models were then compared with the individual ones in order to check that there was no great loss in accuracy. These 3 first where a, β, γ and ξare constrained param- steps only used the data from the first 2 sub- > 0, β < 0, y< 0 and eters: a samples. Fourth step: We used the data of the third sub- sample to validate the model and then put the 3 data sets together and re-estimated parameters for a final global model.
This model has the following properties independent parameters (ie 4 basic param- the model and its first-order (see fig 2): a) by equation 5), estimates of β eters related derivative are continuous; b) α/H is the derived from the estimates of a and; &xi were slope of the DBRMAX over the X curve at 3 shows how by using equation (5). Figure the top of the tree (ie a is the slope of the the model fits to the data for 2 different DBRMAX over the XR curve): α/H is there- trees (a relatively good and a relatively bad fore related to the geometry of the top of fit). For the worst fit, the model slightly un- the crown; c) X ξ.H is the distance be- derestimates the greatest diameter and 0 = tween the top of the stem and the location there is a small discrepancy between the of the thickest branch; d) the thickest observed and predicted locations of the branch of the stem has a predicted value thickest branch. noted Max (DBRMAX): Construction of a single global model At first, we tried to predict the estimated values of Max(DBRMAX) and(ie the di- This model was fitted independently for ameter and the location of the thickest each tree. Since the model contains only 3 branch of the i th tree). Among various
a was not significantly different from 0; 0 this parameter was therefore removed in further analysis. Since the best prediction of Max (DBRMAX) was not as good, we decided to incorporate equation (7) into the individ- ual models by substituting for ξ. We then reestimated the parameters a and y of model (4) in order to investigate the possi- ble relationships between a and y and to predict these parameters by using the whole-tree parameters (β was not directly estimated but was deduced from a and; &xi by using equation 5). Among various combinations, the best equations were: (Statistics of fit: R2= 0.96; RMSE = 0.012; (Statistics of fit: R 2 = 0.77; The regression expressions of ξ, a and y (eq 7, 8 and 9) were then introduced in the individual models to form a global mod- el which was estimated simultaneously for all the trees of the first 2 subsamples. After some modifications due to high correla- tions between some parameters, the mod- el form was: combinations of 1, 2, 3 or more whole-tree descriptors, the best fit for ξ was given by: 2 1 0 ξ =a +R Ca (7) (Statistics of fit: R2= 0.73; RMSE 5.4% = (root mean squared error); P > F =0.001)
(Statistics of fit for 699 observations and els (RMSE 0.32 cm for model 4 vs = 26 trees: RMSE 0.36 cm; P > F RMSE 0.36 cm for model 10), the value = = = of the F statistic was fairly high (F= 3.69) 0.0001) according to the high degrees of freedom The parameter values and their stan- (ie 70 and 621). Thus it appeared that the dard errors were estimated as follows in global model was slightly but significantly table I. less accurate than the set of individual The 2 estimated asymptotic correlations models and that a part of the within- and among parameter estimates with the high- between-tree variation of branch size could est absolute value were: r (a a -0.95 ,) = 86 not be predicted by the tested whole-tree r (a a -0.75. ,) = 78 descriptors and by the relative distance to the top of the tree. Comparison between the tree-by-tree model and the overall model VALIDATION Although the hypotheses necessary for its application are likely to be at least partially the Validation third subsample on violated (there is a within-tree autocorrela- tion and the within-tree error is not rigor- checked how the global model At first, ously homoscedastic) we used an F statis- we 26 trees pre- (10) previously adjusted tic to test the loss of precision between on dicted the DBRMAX distribution for the 60 models (4) and (10). We noted SSE, the trees of the validation sample (ie we used sum of squared residuals, obtained after the parameter values given above). The the nonlinear adjustments: the sum of SSE difference between actual and simulated for the 26 individual models was: 64.0 values (observed DBRMAX minus predict- (with 621 degrees of freedom); SSE for DBRMAX) and the square of this differ- the overall model was: 90.6 (with 691 de- ed calculated for each observation grees of freedom). ence were (a total of 1 728 observations). We ob- Although the root mean squared error tained the following results: was not very different between the 2 mod- the mean difference was -0.229 cm, - which indicates that the model overesti- mated limbsize for the validation sample; differences the of squared was sum - 771.68, which gives root mean squared a difference equal to 0.66 cm which is con- siderably higher than the RMSE obtained for the 26 trees of the first two samples. Global fit of the same model with all tree subsamples The root mean squared error for the 2 427 observations and the 86 trees was: 0.49
The parameter values and their stan- The global model was then reestimated cm. dard errors were estimated in table II. using these equations; it provided a root mean squared error equal to 0.49 cm. The estimated asymptotic correlation among parameter estimates with the high- est absolute value was: r (a a -0.73. ,) = 67 Development of a global model for the 3 subsamples Improvement of the global model The model obtained in Improvement of the for the third subsample global model for the third subsample above was finally adjusted to the 2 427 ob- Using the same strategy as described in servations coming from all 86 trees. The Construction of a single global model for root mean squared error was 0.47 cm with the 60 trees of the third subsample we first the following parameter values (since b 4 obtained: and bwere not significantly different from 11 zero, these parameters were removed) (table III). The estimated asymptotic correlation among parameter estimates with the high- est absolute value was: r (b b -0.82. ,) = 56 The fit of this model for 2 different trees is illustrated in figure 4. If adjusted to the 26 trees of the first 2 subsamples, this model provides a root mean squared error equal to 0.37 cm which is fairly similar to the 0.36 cm given in Construction of a single global model. Thus this last model was considered as the best compromise for the whole data set.
DISCUSSION is very restricted that their growth is so nearly stopped, and the ground they near dead. are Biological interpretation Consequently, the first part of the model with a curvilinear form predicts limbsize from the tip of the stem to approximately The predominant effect of the distance the base of the live crown: qualitatively, the from the tip, also observed and modelled second degree polynomial equation takes by Madgwick et al (1986), Maguire et al into account the intrinsic geometry of the (1990, op cit)) is actually the result of dif- crown as well as the beginning of the ef- ferent complementary aspects: fects of the mutual inter-tree shading. The softwood species present a conical - second part of the model which is also a crown, due to a strong apical dominance; second degree polynomial describes the the effect of the age of the branch: older - part of the crown that goes from the base branches are located far away from the tip; of the live crown to the dead branches. at a certain distance from the tip, the - The estimated values of a b and ,, b 11 2 branches belong to the part of the crown indicate that the thickest 3 b parameters where mutual inter-tree interference oc- branch seems to be actually located higher curs (shading and stress marks); than the base of the living crown (eg a 1 = further down, the branches belong to the 0.56 in Construction of a single global - model). Since the maximum of the curve is part of the crown where sunlight exposure
generally quite flat, there isa wide portion between the height to the base of the ence of the stem where maximum limbsize per live (as previously defined) and the crown whorl is nearly constant. However, this height to the first five branch; the thickest point should be analysed further to check branches are located nearer to this latter whether the difference between ξand base height. of the living crown is due to an inadequacy For the first 2 samples, the crown ratio of the model or to an early effect of the CR 2 (ie the ratio 100.(H- HC)/H) was the competition that precedes crown reces- best predictor. When considering this re- sion. duced data set the weight of the trees be- Concerning the overall model estab- longing to the AFOCEL stand is high lished for the 3 subsamples (see Develop- (16 trees / 26 trees) in the regression anal- ment of a global model for the 3 subsam- ysis. Since this stand is more homogene- ples), we noticed: 1) a slight over- ous (ie the total heights of the trees are estimation of the DBRMAX for the smallest very similar) and the slope is gentle, the trees (ie for most trees which have a DBH crowns are nearly symmetrical and have a < 16 cm; and 2) a slight but systematic un- regular external shape; hence, the differ- derestimation for the trees which are locat- ence between CR 2 and CR 3 does not ed in edge conditions or in stands installed vary much from one tree to another. on sites with steep slopes. This is probably Therefore all these remarks seem to be due to the fact that the standard whole-tree consistent. The distribution of the maxi- measurements introduced in the model mum limbsize per annual growth unit along cannot take into account the relative over- the stem appears to be sensitive to the development of the branches that are symmetry of the crown and to the sunlight oriented towards the best sunlight condi- exposure conditions. tions. Moreover the model underestimated Comparison with other models but frequently the maximum limb- slightly size for the trees of the AFOCEL stand. This is not really surprising since: 1),the Maguire et al’s model (Maguire et al, 1990, weight of these trees in the whole data set op cit) focuses on young Douglas fir trees is relatively small; and 2), they belong to a from plantations before crown closure and, stand which has been submitted to a more hence, where the base of the live crown is intensive silviculture than the others (ie the very near to ground level. The shape of spacing conditions of these trees have their model is curvilinear rather than linear been more favourable to their growth). from tip down to stem base. This is consis- Again, it is likely that the model does not tent with the fact that, even without inter- reflect their increased exposure to sunlight. tree competition for light, the growth of the lower branches is reduced (Mitchell, 1975). The crown ratio CR 3 and the height to Due to younger ages and the open- the first live branch (HFLB) turned out to be the best crown parameters when we grown condition of Maguire et al’s trees, it tried to validate the model. This is probably is difficult to compare their results with due to the fact that the proportion of trees ours. However it is important to note that located in stands with steeper slopes their model does not separate the within- (> 20 °C) is higher in this part of the data and between-tree variabilities, since the di- mensions of the trees are not taken into set. Steep slopes introduce an asymmetry in the crown and produce a greater differ- account. This might at least partially ex-
plain the great variance around their mod- of the absence of a good definition cause el and why our first attempts (not reported in terms of limb size and insertion angle, here) to model branch size variation along and because their occurrence cannot be the stem without including whole-tree de- predicted with deterministic models. scriptors were not conclusive. Vertical distribution of branch diameter Vertical distribution of branch diameter and genetic origin and growth conditions On different families of a Polish prove- nance studied by Van de Sype (personal Site growth conditions (eg site index) are communication), he observed that inde- partially hidden in the model by the use of pendently of growth vigour, branches are relative depth into the crown as an inde- proportionally thicker for certain families pendent variable. To predict the actual than for others. Such differences have also size of the branches, for instance in the been established by Cannell and Bowler merchantable part of the stem, it is neces- (1977) on Picea sitchensis. Our sampled sary to return to the absolute values of trees probably belong to the same genetic depth in the crown which are linked with origin (ie the same provenance): the Gé- height growth and therefore with site con- rardmer provenance. It will therefore be im- ditions. portant to check whether a part of the re- Tree growth conditions are also deter- sidual variability around the model may be mined by the current and initial stand den- attributed to genetic effects. This will be sities, by the silvicultural practices and by done by fitting the model to various prove- the competitive status of the tree. The nances. main effect of the stand management is re- As cited by Schmidt-Vogt (1977) and flected in crown development which is, at also observed by Hakkila (1971),different least partially, included in the proposed patterns of branchiness exist: brush form, model through crown ratio variables. Nev- comb form, flat form, with narrow or wide ertheless, as already observed for widely lateral extension. Do these patterns have a spaced trees (ie AFOCEL stand) or for strong influence on the accuracy of our edge trees, the overall model does not de- model? Using our field notes we were not scribe perfectly the trees submitted to fa- able to establish an actual effect of branch vourable or asymmetrical sunlight expo- form. In fact, only three trees presented sure. comb-shaped branches and these trees The growth conditions at high eleva- were accurately modelled. During future tions imply branch and leader damage sampling, such characteristics will have to which are caused by late frost and snow be noted again. weight. For some trees we indeed ob- served that the model does not describe the peak of the empirical curves very well. Utilization of the model This fact could be explained, at least part- ly, by the occurrence of "ramicorn branch- First, it must be emphasized that the mod- es" that attain greater diameters than other developed in order to predict the el branches. Although these branches are was vertical trend in maximum limb size very important in lumber grading, they mean point of time and that it does not repre- have not been analysed in this study be- at a
sent the dynamics of the branching struc- Forest Survey data) so that CR2 or CR 3 ture (ie branch growth and crown reces- values will have to be estimated from other sion). This point may partly explain the dif- whole-tree descriptors (eg AGE, DBH, H). ference that was observed between ξ and This procedure will probably introduce a the base of the living crown (see Biological major source of variability which has not interpretation section). Above all, it implies been assessed in this study. that the direct application of the model to The model has several other applica- the outputs of a tree growth model may tions as well: lead to some inconsistencies between the for logging operations and for standing or - successive predictions of maximum limb felled tree grading, information about the size at a given height for the same tree. height of the thickest branch or about the One interesting feature of this model is height to a given branch size are very use- that it provides relatively good estimates of ful. For instance, in the Soviet Union (Ar- the maximum branch diameters along the lauskas and Tyabera, 1986) or in Finland stem as well as quantitative indications (Hakkila et al, 1972, op cit; Leban, 1989) about the variability around these predic- the size of the branches combined with the tions. Although the underlying statistical length of the merchantable logs determine assumptions are probably violated, the the quality and value of trees; confidence intervals (see figs 3, 4) provide for pruning, the choice of the tools as - rough estimates of extremes in limbsize. well as the assessment of the costs also As previously stated, a more rigorous sta- require information about the size of the tistical analysis recognizing autocorrelated branches that would be removed by differ- and heteroscedastic errors was outside of ent pruning lifts (Riou-Nivert, personal the scope of this paper and will now be communication); performed. Information about the variability lastly, due to the close links between around the model could then be used in - maximum or mean whorl limbsize and Monte-Carlo simulations to provide pro- branch length, our model could be used to babilistic inputs to SIMQUA rather than predict the external shape of the crown. purely deterministic predictions. The proposed model has been estab- lished for mid-size trees (15 cm ≤ DBH ≤ CONCLUSION 35 cm) in even-aged stands. It cannot be extrapolated to smaller or bigger trees without further validation. Indeed, the be- Since the estimated confidence intervals haviour of the model for bigger trees is un- are relatively broad around the predicted known and the slight overestimation for the limbsize values and since lumber grading smallest trees indicates that the model rules are heavily dependent on the maxi- should be improved for small and young mum limbsize in boards, trees with similar trees. Its application to uneven-aged whole-tree descriptors may actually pro- stands or to steep slopes should also be duce different grades. Thus, the accuracy avoided due to the highly asymmetrical de- of limbsize predictions is crucial when at- velopment of the crown in these condi- tempting to apply such models to opera- tions. tional inventory data to estimate wood product quality. One other practical problem is that Our approach provides into measurement of crown ratio is rarely per- insight an still many im- formed in operational surveys (eg National question, but there this are
of forest stands, trees and terrain in portant points to be addressed: 1),the im- Sweden) Stud For Suec 20 provement of the accuracy of the pro- posed Arlauskas LS, Tyabera AP (1986) Branchiness model by taking into account more of stems in Norway spruce forests in Lithua- precisely the effects of site and silvicultural nia. Lesnoï Zh 1, 13-16. treatments; 2), the analysis of the genetic Cannell MGR, Bowler KC (1977) Spatial ar- variability of limbsize distributions; 3), a rangement of lateral buds at the time they more rigorous statistical analysis of the re- form on leaders of Picea and Larix. Can J gression models; 4), the proposal of pro- For Res 8, 129-137 babilistic simulation procedures that use De Champs J (1989) Effet de la densité de plan- the information provided about the residual tation sur la croissance en diamètre, la forme variability around the model; 5), and a dy- et la branchaison du Douglas. Ann AFOCEL namic approach of branching structure 1988, 232-283 that would allow the establishment of a di- ENGREF, INRA, UCBL (1990) Modélisation de la Croissance et de la Qualité du Bois de rect and consistent link with growth and l’Épicéa Commun : Objectifs, Méthodes et yield models. Premiers Résultats. ENGREF (Nancy), Doc Interne, october 1990, 42 pp Hakkila P (1971) Coniferous branches as a raw ACKNOWLEDGMENTS material source. Commun Inst For Fenn 75- 1, 60 pp This work was partially supported by two grants Hakkila P, Laasasenaho J, Oittinen JK (1972) from the French Ministry of Agriculture and Fo- Branch data for logging work. Folia For (Hel- rests. The authors are grateful to JF Dhôte (La- sinki) 147, 15 pp boratoire ENGREF/INRA de Recherches en Jacamon M (1983) Arbres et Forêts de Lorraine. Sciences Forestières, Nancy) and G Nepveu SAEP, Colmar Observations (Station de Recherches sur la Qualité du Bois) Kärkkäinen M (1972) on the for reviewing the manuscript, to C Houssement, branchiness of Norway spruce. Silva Fenn 6 P Michel, J Perrin, A Perrin, C Herbé and P Gel- (2), 90-115 haye (Station de Recherches sur la Qualité des Leban JM (1989) Compte-Rendu de Mission en Bois, INRA, Nancy) and H Joannès (Station de Finlande, INRA, 01/04/1989-15/04/1989. Génie Logiciel, INRA, Nancy) for technical as- Mission No 634/89 sistance. Leban JM, Duchanois G (1990) SIMQUA : un They also wish to thank the Office National logiciel de simulation de la qualité des bois. des Forêts (ONF) and the Association Forêt- Ann Sci For 47 (5), 483-493 Cellulose (AFOCEL) for their authorization to Madgwick HAI, Tann CO, Fu Mao-Yi (1986) fell and/or measure their trees. They also are Growth development in young Picea Abies deeply grateful to D Maguire (College of Forest stands. Scand J For Res 1, 195-204 Resources, University of Washington, Seattle) n Madse TL, Moltensen P, Olesen PO (1978) and another anonymous reviewer for their help- The influence of thinning degree on basic ful comments on the first version of the paper. density, production of dry matter, branch thickness and number of branches of Norway spruce. Forstl Forsøgsvaes Serv Dan 36 REFERENCES (H.2.22), 183-203 Maguire D, Hann D (1987) A stem dissection technique for dating branch mortality and re- Ager BH, Nilsson NE, von Segebaden G (1964) constructing past crown recession. For Sci Beskrivning av vissa skogstekniskt betydel- 33 (4), 858-871 sefulla bestands- och trädegenskaper samt Maguire D, M&oelig;ur M, Bennett WS (1990) Simu- terränförhallanden (Description of some for lating branch diameter and branch distribu- logging operations important characteristics
Tomassone R, Lesquoy E, Millier C (1983) La Douglas fir. IUFRO World tion in young Régression, Nouveaux Regards Sur Une An- Congr Montreal, Quebec, Canada, August 9, cienne Méthode Statistique. Masson, Paris, 1990 180 pp Max TA, Burkhardt HE (1976) Segmented poly- (1985) The quality and value of nomial regression applied to taper equations. Uusvaara O For Sci 22, 283-289 goods from plantation-grown Scots pine. sawn Commun Inst For Fenn (Helsinki) 130, 53 pp Mitchell KJ (1975) Dynamics and simulated yield of Douglas fir. For Sci Monogr 17, 39 pp Western H (1971) Tre- og Kvistdata (Tree and branch study). Norske Skogsforsoksvesen Schmidt-Vogt H (1977) Die Fichte. Paul Parey, Driftsteknisk Rapp No 10, 83-93 Hamburg, 3 vol